
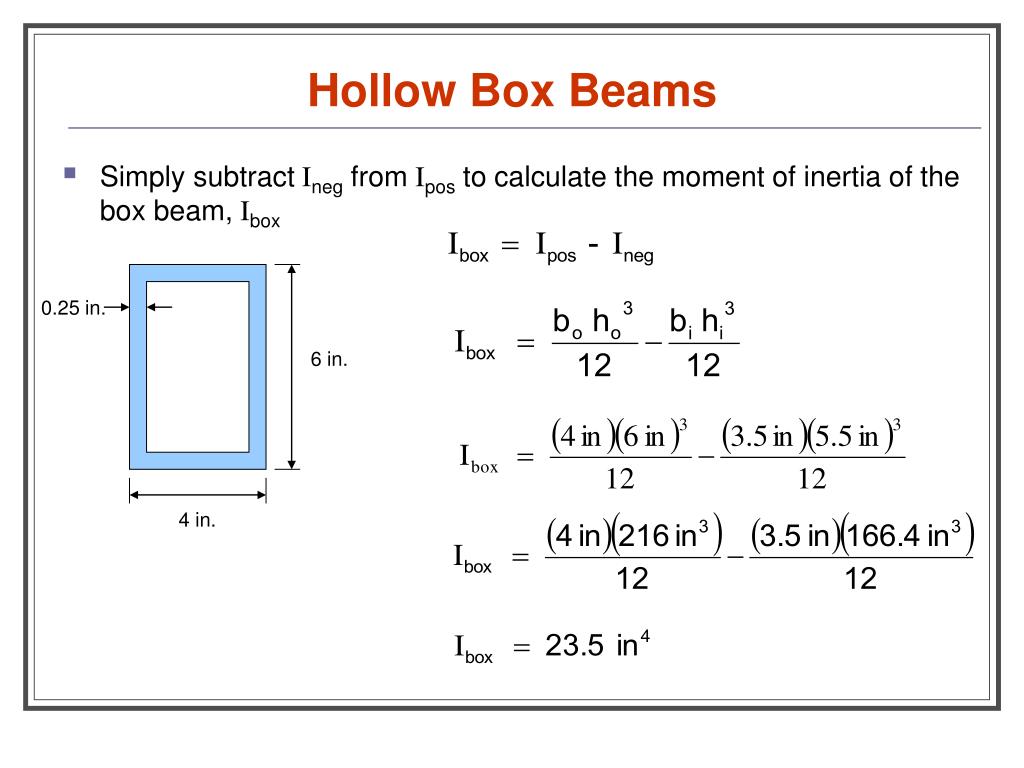
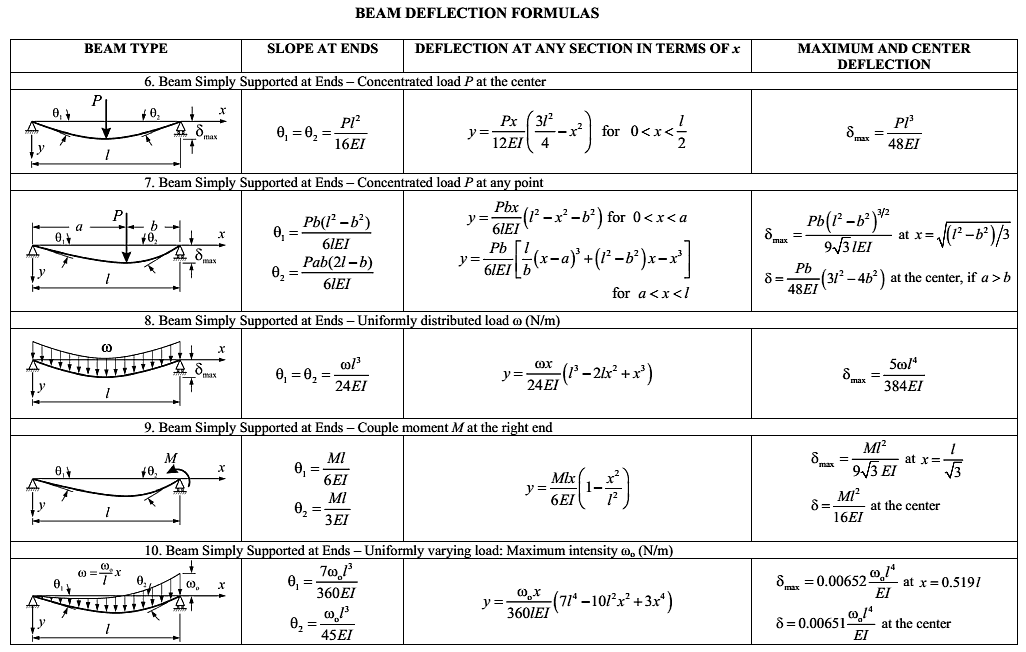
The units of beam deflection formula are expressed in terms of force, length, moment of inertia or elasticity modulus.Based on the location of application of force, cantilever and simply supported bemas can be further classified.Deflection in beams can be broadly classified as: Cantilevers and Simply Supported Beams.Deflection Consideration: The maximum deflection of a loaded beam should be within a certain limit so that the strength and efficiency of the beam should not be affected.The deflection at any point x, along the span of a uniformly cantilevered beam can be calculated using:
#Cantilever beam moment of inertia formula free#
The deflection, at the free end B, of a cantilever beam under a uniform load is given by:
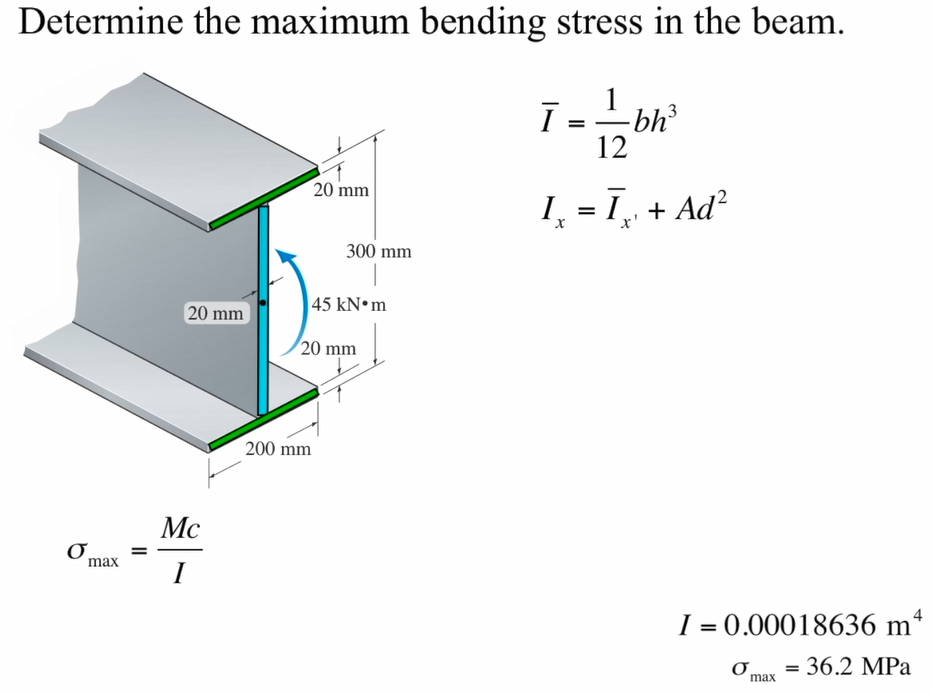
Uniformly-loaded cantilever beams have the force acting uniformly through out the length of the beam. When x = L (the end of the beam), δx is identical to δB in the equation above. The deflection at any point x, along the span of an end loaded cantilever beam can be calculated using: If the span doubles, the deflection increases eightfold. To calculate the deflection of the cantilever beam with force at free end we can use the below equation: Following is a schematic to understand this a little better. In this case of cantilever beams, the load is applied at a single point on the beam. Cantilever beams are further classified as: These types of objects would naturally deflect more due to having support at one end only, so that the slope and deflection at that end must be zero. The formulas expressed are approximations developed for long, slender, homogeneous, prismatic beams with small deflections and linear elastic properties.Ĭantilever beams are the special types of beams that are constrained by only one given support. Important Topics Related to Beam Deflection Formulaīeams can vary greatly in their geometry and composition.


 0 kommentar(er)
0 kommentar(er)
